
Mathematical criminology
Crime is rarely spatially or temporally constant and often occurs in spikes (in time) or hotspots (in space). The development of mechanistic dynamical models that can predict these spikes and hotspots is an emerging area in the field of mathematical criminology, where we develop a range of agent-based and partial-differential equations-based models to analyse the qualitative behaviour of crime and combine it with data. This work requires an interesting combination of mathematical modelling, dynamical systems analysis and Bayesian statistics.
This area of research is linked to the Centre for Criminology at the University of Surrey.
Research leads

Professor David Lloyd
Professor

Dr Naratip Santitissadeekorn
Senior Lecturer
Modelling community and crime interaction

Understanding the formation of crime hotspots and their interaction with the community requires the development of continuum models that capture various criminological theories such as repeat victimisation, collective efficacy and social disorganisation. Such models can then be analysed using a variety of mathematical techniques from dynamical systems theory and numerical methods.
This area of work also looks at the interaction of these behaviours with the police, uncovering the phenomenology of when crime hotspots use a range of dynamical systems and PDE techniques.
This project, led by David Lloyd, is in collaboration with Ian Brunton-Smith (Department of Sociology, Surrey).
Modelling crime escalation and desistence

New data is becoming available which allows researchers to investigate why people desist from, or persist in, a life of crime – an area of work that has major implications for policy making and policing. This project aims to carry out a data analysis and develop continuum models to understand and quantify transition rates from/to crime.
Designing continuum models that incorporate explanatory variables and stratified data (such as age/location) will help explore various interventions and assess their impact. This project, led by David Lloyd, is in collaboration with Ian Brunton-Smith (Department of Sociology, Surrey).
Data assimilation methods for crime forecasting and influence networks
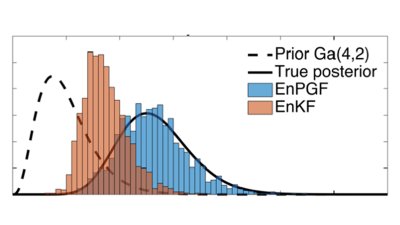
David Lloyd and Naratip Santitissadeekorn are developing novel methods to combine data and models to improve short-term crime forecasting, with a focus on helping victims.
This work combines dynamical systems models with Bayesian statistical methods to help quantify our uncertainty when making decisions.
An interesting application of these methods is to detect dynamic social influence in communication networks and gang violence. The project is a collaboration with Martin Short (Georgia Tech).